
Section 1:
![]() 2 x 2 Tables
2 x 2 Tables
![]() Chi-Square
Chi-Square
Degrees of Freedom
p-Value
Flip Coin 100 times
Breast Cancer Example
Null hypothesis
The null hypothesis is that there is no difference between coin A and coin B in the probability of tossing a heads (or tails). This hypothesis can be tested. The test on a 2 x 2 table may be carried out by a convenient formula.
The test is a two-step process
- First,
one calculates the so-called chi-square statistic (symbolized by
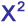 and
pronounced as kai-square) and the degrees of freedom for the table.
and
pronounced as kai-square) and the degrees of freedom for the table.
- Second,
one uses the
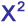 statistic
to look up (in a table or computer program) the probability–called
the p-value–of
an outcome as extreme or more extreme than the observed outcome
occurring by chance.
statistic
to look up (in a table or computer program) the probability–called
the p-value–of
an outcome as extreme or more extreme than the observed outcome
occurring by chance.
We will reject the null hypothesis if the p-value is equal to or less than the conventional requirement for statistically significant results, which is set at the so-called significance level of 0.05, or 5%.
Step 1-A: calculate chi-square
To illustrate, if a, b, c, and d are the numbers in the cells of the
2 x 2 table (a, b in the first row and c, d in the second row), then ![]() may
be calculated as follows:
may
be calculated as follows:
|
|

|
|
|
|
In this case, the calculation yields . . .
![]() =
=  =
0.8333
=
0.8333
